Fraction. Multiplication of common fractions, decimals, mixed

In the course of middle and high school students took the theme “Fraction”. However, this concept is much broader than is given in the learning process. Today the concept of fractions is not uncommon, and not everyone can perform calculations of any expression, for example, multiplying fractions.

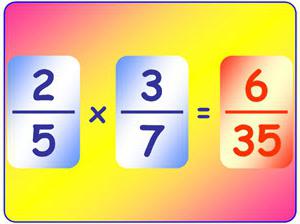
What is a fraction?

Historically, that fraction appeared because of the need to measure. As practice shows, often there are examples on the determination of the length of the segment, volume of a rectangular parallelepiped, the area of the rectangle.

Initially, students are introduced to a term such as share. For example, if you divide the watermelon into 8 parts, then each will get one eighth of a watermelon. This is one part of the eight and is called a share.

The Percentage equal to ½ of any magnitude, is called a half; ⅓ - a third; ¼ - a quarter. Entries of the form ⁵/₈, ⁴/₅, ²/₄ is called common fractions. Common fraction divided into the numerator and denominator. Among them is a hell of a fraction, or fraction. Slash can be drawn in horizontal and oblique lines. In this case, it denotes a division sign.

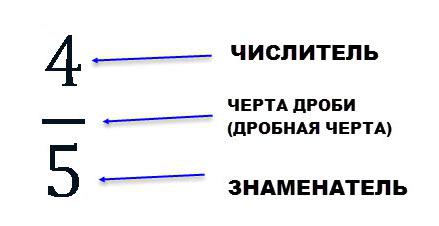
The Denominator represents how many equal shares share value, the subject; and the numerator – how many equal shares are taken. The numerator is written above the fraction bar, the denominator under it.

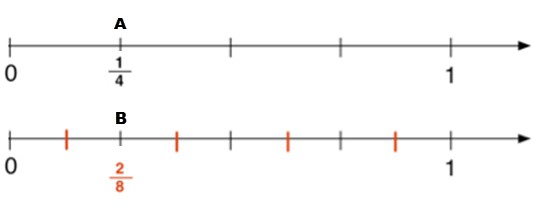
The only show fractions on the coordinate of the beam. If a single segment divided into 4 equal shares, to designate each share of Latin letter, then you can get a great visual aid. So, point a shows the percentage equal to ¹/₄ from only a single cut, and the point In the notes ²/₈ from the current segment.

Recommended

"Knowledge is light and ignorance is darkness": the value, meaning and alternatives

There are some sayings that would seem to need no explanation, such as “teaching & ndash; light and ignorance – darkness”. But some still do not understand their meaning. But not only for such people is written by our article. I...

What was invented by Mendeleev for the army. The history and fate of the invention

D. I. Mendeleev was a brilliant Russian scientist-polymath, who made many important discoveries in various fields of science and technology. Many people know that he is the author of “Fundamentals of chemistry" and the periodic law of chem...

The origin of the Slavs. The influence of different cultures

Slavs (under this name), according to some researchers, appeared in the story only in 6 century ad. However, the language of nationality bears the archaic features of the Indo-European community. This, in turn, suggests that the origin of the Slavs h...

Types of fractions

There are common Fractions, decimals, and mixed numbers. In addition, fractions can be divided into right and wrong. This classification is more suitable for common fractions.

Under proper fraction understand the number that has the numerator less than the denominator. Accordingly, the improper fraction-a number that has a numerator greater denominator. The second kind usually written as a mixed number. Such an expression consists of a whole part and a fractional part. For example, 1½. 1 - the whole part, ½ - fractional. However, if you want to do some manipulations with the expression (division or multiplication of fractions, their reduction or transformation), translates a mixed number to an improper fraction.

The Right of the fractional expression is always less than unity, while the wrong – greater than or equal to 1.

As for the decimals, under such an expression record, which presents any number that the denominator of the fractional expression of which can be expressed through a unit with a few zeros. If the fraction is correct, then the integer part of the decimal will be zero.

To record a decimal, first write the integer part, separate it from the decimal with a comma and then write the fractional expression. It must be remembered that after the decimal point the numerator must contain the same number of numeric characters, how many zeros in the denominator.

Example. Represent the fraction 7²¹/₁₀₀₀ in decimal.

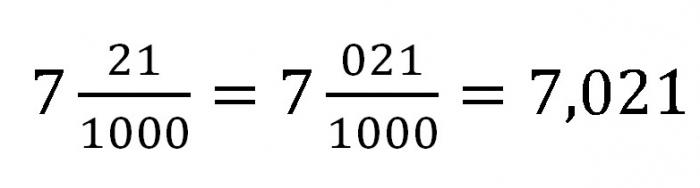
The translation Algorithm improper fractions to mixed number and Vice versa

Write the answer an improper fraction incorrectly, so it should convert to a mixed number:

• Split the numerator into the denominator available;
• In the specific example of an incomplete private – whole;
• Balance – the numerator of the fractional part, the denominator remains unchanged.

Example. Translate the improper fraction to a mixed number: ⁴⁷/₅.

Solution. 47 : 5. Partial quotient is equal to 9, remainder = 2. So, ⁴⁷/₅ = 9²/₅.

Sometimes you need to represent a mixed number as improper fractions. Then you need to use the following algorithm:

• The integer part is multiplied by the denominator of the fractional expression;
• The product is added to the numerator;
• The result is written in the numerator, the denominator remains unchanged.

Example. To represent a number in mixed form as improper fractions: 9⁸/₁₀.

Solution. 9 x 10 + 8 = 90 + 8 = 98 &- the numerator.

Response: ⁹⁸/_10.

Multiplication of ordinary fractions

Over ordinary fractions you can perform various algebraic operations. To multiply two numbers, you need to multiply the numerator with the numerator and the denominator with the denominator. And multiplication of fractions with different denominators Is no different from the works of fractional numbers with equal denominators.

It Happens that after finding the result of the need to reduce the fraction. It is imperative to simplify the resulting expression. Of course, we cannot say that the improper fraction in the answer – this is a mistake, and call the correct answer it too difficult.

Example. Find the product of two fractions: ½ and ²⁰/₁₈.

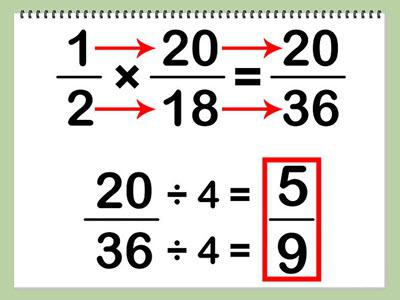
As you can see, after finding the writing appears cancelable fractional entry. And the numerator and denominator in this case is divided into 4, and the result is the response ⁵/₉.

Multiplying fractions decimal

The Product of decimals is quite different from the ordinary works on his principle. So multiplying fractions is:

• Two decimals you need to write to each other so that the rightmost digits appeared one below the other;
• You need to multiply the numbers, despite the comma, that is, as natural;
• Count the number of digits after the comma in each of the numbers;
• In the resulting after multiplication the result should count the right as many numeric characters, how much is contained in the sum of both multipliers after the comma, and put a dividing sign;
• If the figures in the piece were less, then they need to write as many zeroes to cover that number, a comma, and attribute to the integer part equal to zero.

Example. Calculate the product of two decimals: 2.25 to 3.6.

Solution.

Multiplication of mixed fractions

To compute the product of two mixed fractions, we need to use the rule of multiplication of fractions:

• Convert the numbers mixed to improper fractions;
• Find the product of the numerators;
• Find the product of the denominators;
• Record the result;
• Simplify expression.

Example. Find the product of 4½ and 6²/_5.

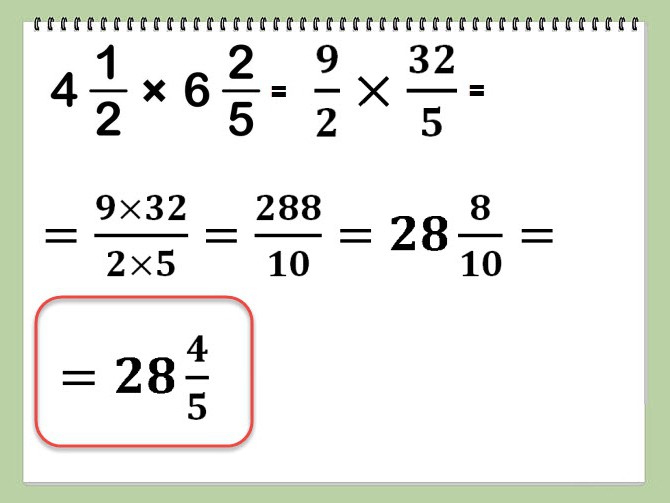
Multiply numbers in the fraction (fractions in number)

In Addition to finding the product of two fractions, mixed numbers, there are jobs where you need to multiply a natural number by a fraction.

So, to find the product of decimals and natural numbers, need:

• Record the number under the fraction so that the rightmost digits appeared one above the other;
• Find work, despite the comma;
• In the result to separate the integer part from the fractional with the decimal point, counting to the right the number of characters that is the decimal point in the fraction.

To multiply a fraction by an ordinary number, you should find a product of a numerator and a natural multiplier. If the answer is cancelable fraction, it should be converted.

Example. Calculate the product ⁵/₈ and 12.

Solution. ⁵/₈ * 12 = ^(5*12)/₈ = ⁶⁰/₈ = ³⁰/₄ = ¹⁵/₂ = 7¹/_2.

Response: 7¹/_2.

As you can see from the previous example, it was necessary to reduce the result and convert the improper fraction expression into a mixed number.

Also multiplication of fractions applies to finding the works of a number in mixed form, and natural factor. To multiply these two numbers, the integer part of the mixed multiplier is multiplied by a number the numerator is multiplied by the same value, and the denominator remains unchanged. If you want, you need to simplify the result.

Example. Find the product of 9⁵/₆ and 9.

Solution. 9⁵/₆ x 9 = 9 x 9 + ^{(5 x 9)}/₆ = 81 + ⁴⁵/₆ = 81 + 7³/₆ = 88¹/_2.

Response: 88¹/_2.

Multiplication by the multipliers of 10, 100, 1000 or 0,1; 0,01; 0,001

Of the previous paragraph leads to the following rule. To multiply decimal fractions by 10, 100, 1000, 10000, etc. you need to move the decimal point to the right by that number of characters number of digits as zeroes in the multiplier after one.

Example 1. Find the product of 0.065 and 1000.

Solution. 0,065 x 1000 = 0065 = 65.

Response: 65.

Example 2. Find the product of 3.9 and 1000.

Solution. 3.9 x 1000 = 3,900 x 1000 = 3900.

Response: 3900.

If you want to multiply a natural number and 0,1; 0,01; 0,001; 0,0001, etc., should be moved to the left of the decimal point in the resulting work on as many symbols of numbers, how many zeros is set to one. If necessary, prior to a natural number writes zeros in sufficient quantity.

Example 1. Find the product of 56 and 0.01.

Solution. 56 x 0.01 = 0056 = 0,56.

Response: 0,56.

Example 2. Find the product of 4 and 0.001.

Solution. 4 x 0.001 = 0004 = 0,004.

Response: 0,004.

So, finding the pieces of different fractions should not be a problem, except that the counting result; in this case, without a calculator simply cannot do.