The types of matrices. A step matrix. Bringing the matrix to the step and triangular form

Matrix is a special object in mathematics. Portrayed in the form of a rectangular or square table, which is composed of a number of rows and columns. In mathematics there are a large variety of matrices that differ in size or content. The number of rows and columns are referred to as orders. These objects are used in mathematics to organize your record systems of linear equations and easy search results. Equations using the matrix are solved by the method of Carl Gauss, Gabriel Cramer, minors and algebraic additions, as well as many other ways. Basic skills in working with matrices is to bring to the standard view. But first let's see what types of matrix isolated mathematics.

The Null type

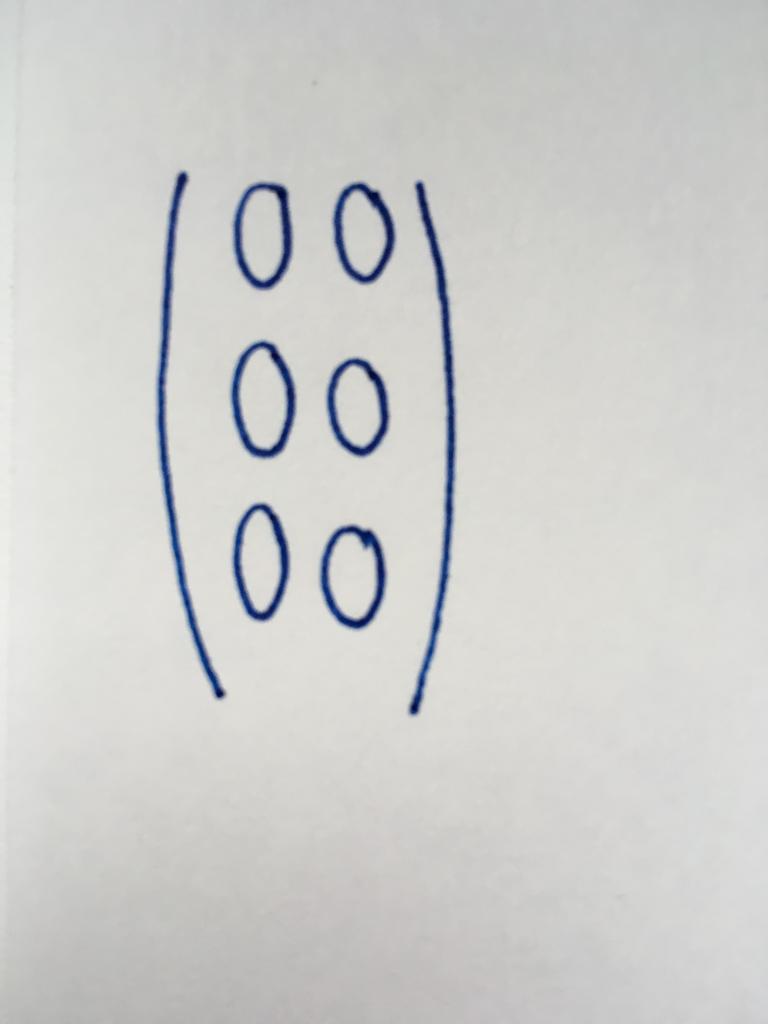
All the components of this matrix are zero. Meanwhile, the number of its rows and columns are totally different.

Square type

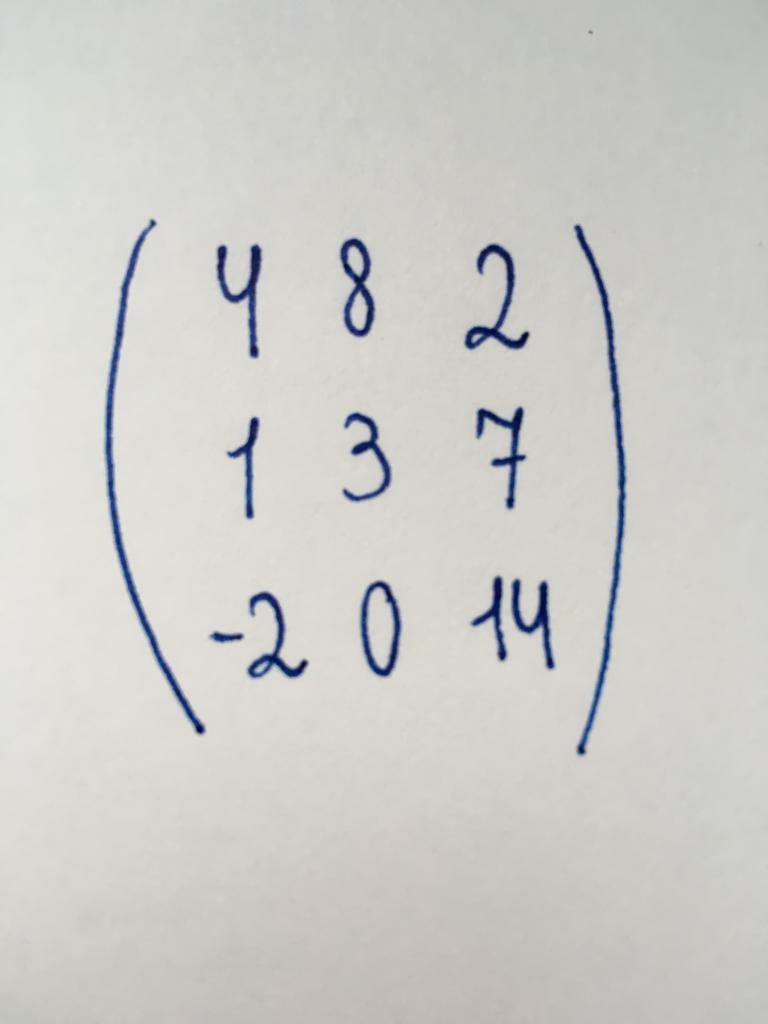
The Number of columns and rows of this matrix is the same. In other words, it is a table of the form "square". The number of its columns (or rows) are called the order. Special cases considered the existence of the matrix of the second order (matrix 2x2), fourth order (4x4), ten (10x10), seventeenth (17x17), and so on.

Vector-Stober

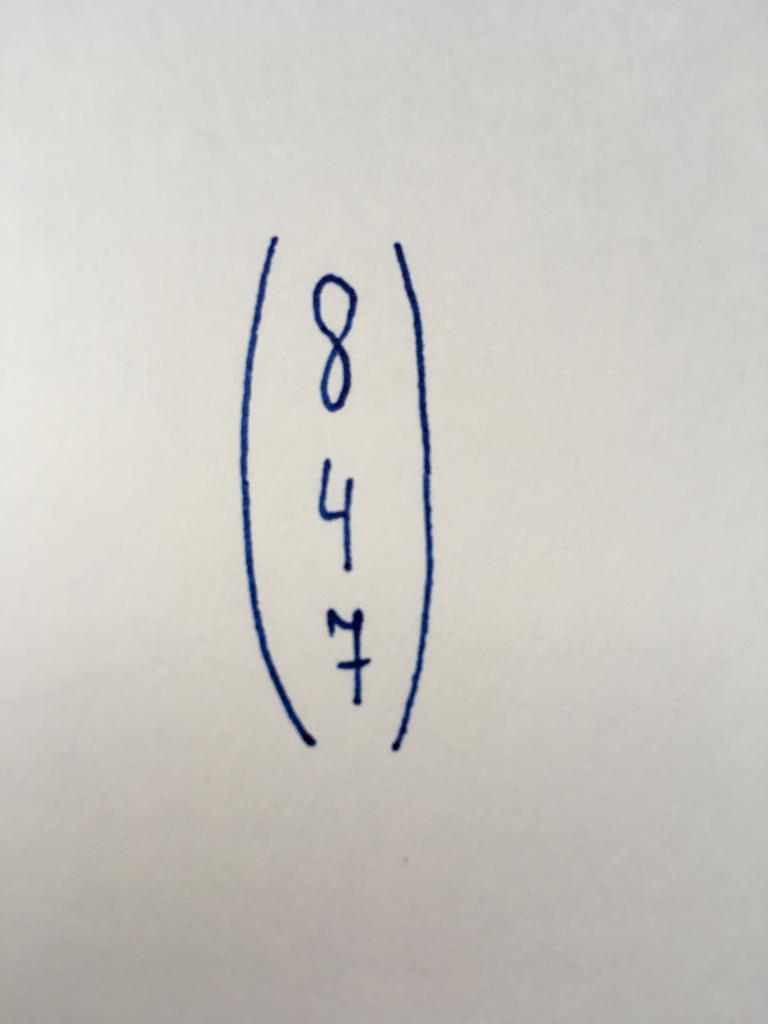
This is one of the simplest types of matrix containing only one column, which consists of three numeric values. It represents the number of free members (numbers that are independent variables) systems of linear equations.

Vector-line

Similar to the previous one. Consists of three numerical elements, in turn arranged in a single row.

Diagonal

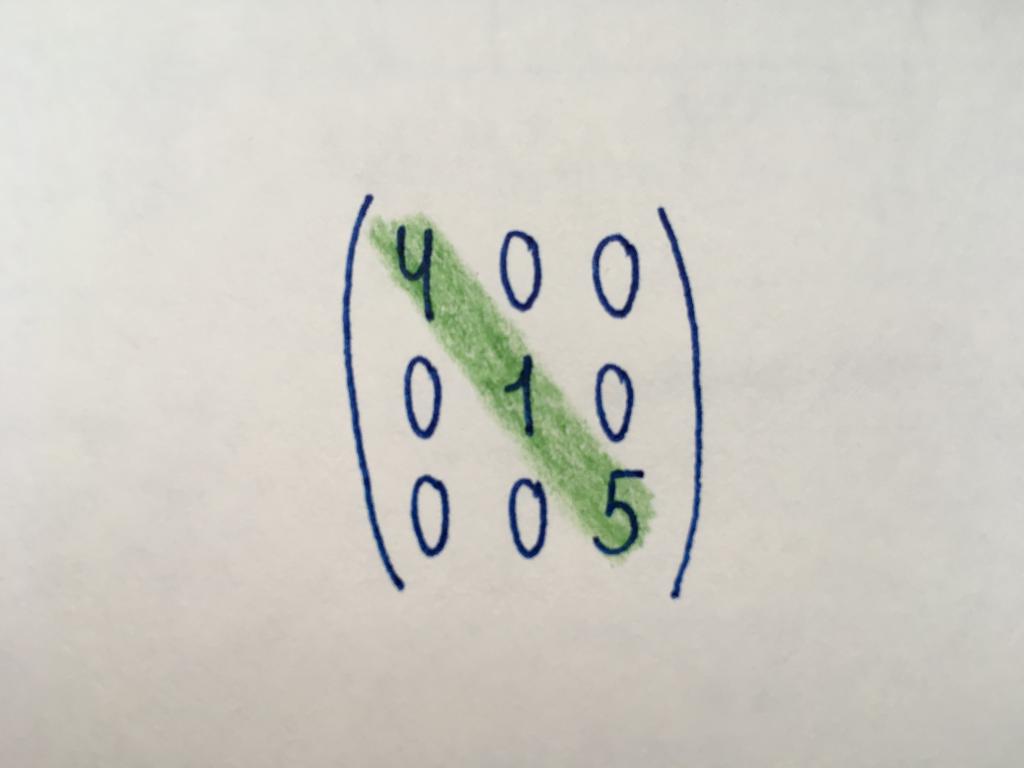
The Numeric values in a diagonal matrix taking only the components of the main diagonal (highlighted in green). The main diagonal starts with the element at the top-right corner and ends in the third column of the third row. The remaining components equal to zero. Diagonal type only represents a square matrix of any order. Among the diagonal matrices, there are scalar. All of its components take the same value.

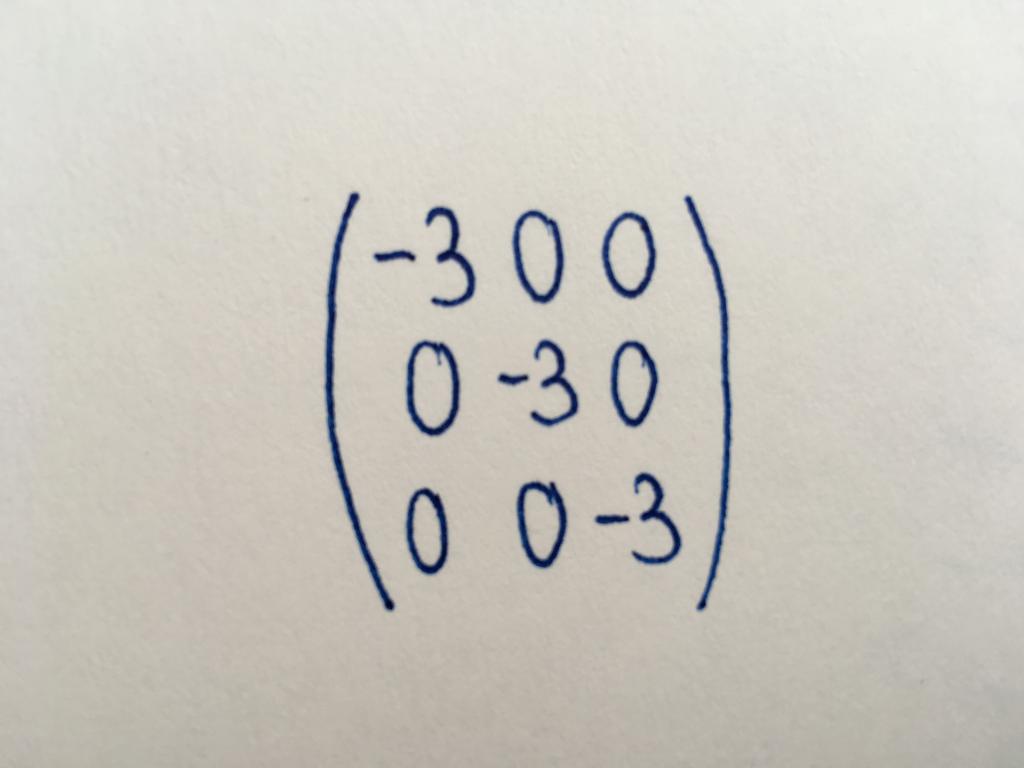
Identity matrix

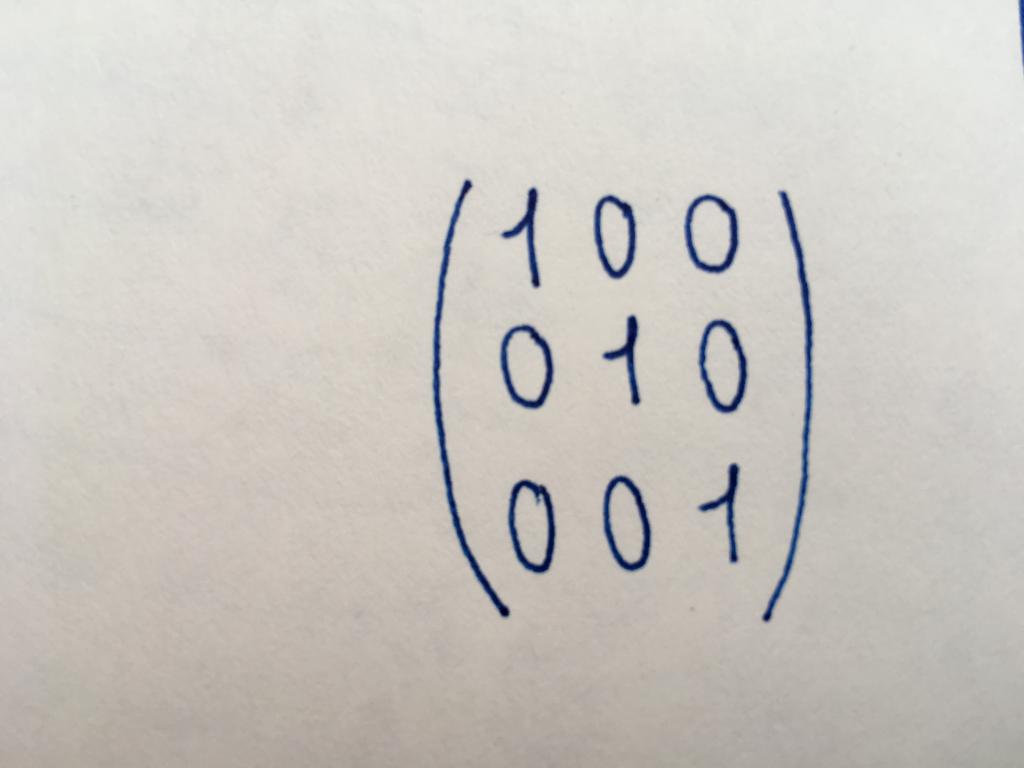
A subspecies of the diagonal of the matrix. All its numerical values are units. Using a single type of matrix tables, perform base conversions, or find the inverse of the source.

Canonical type

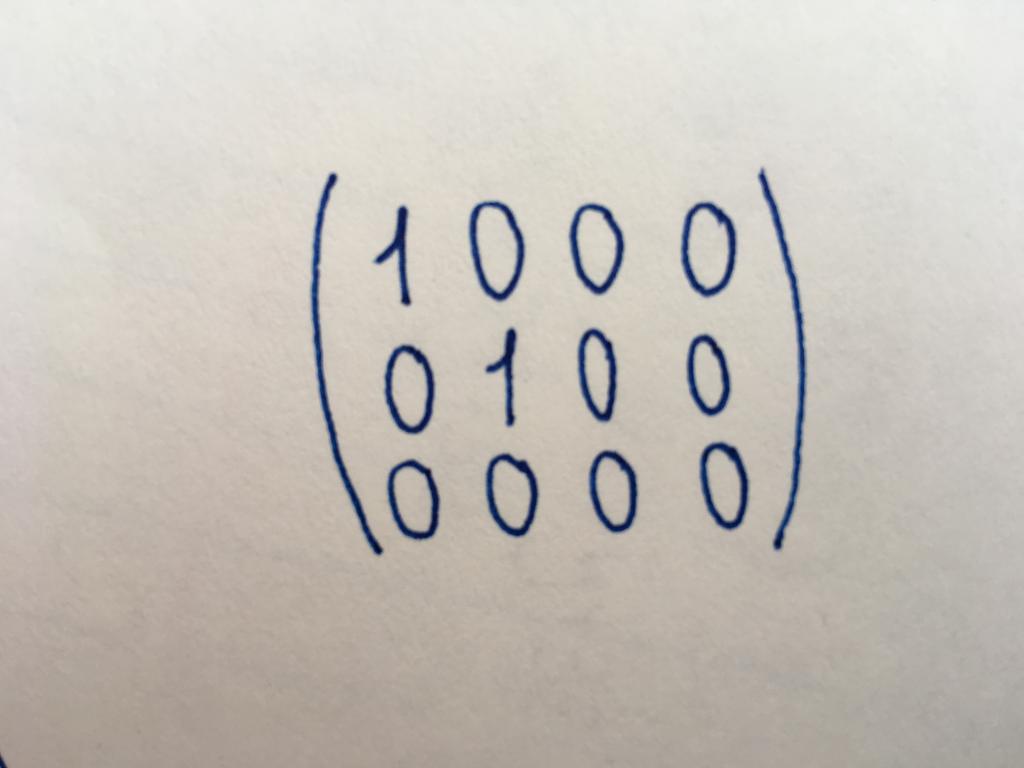
The Canonical form of the matrix is one of the main and adjustment is often necessary. The number of rows and columns in the canonical matrix are different, they do not necessarily belong to the square type. It is somewhat similar to the identity matrix, but in her case not all components of the main diagonal are set equal to one. Pavlovianly units may be two, or four (depending on the length and width of the matrix). Or units may not be available at all (when it is zero). The remaining components of the canonical type as the elements of the diagonal and a single zero.

Triangular

One of the most important types of matrix used in the search for its determinants and in the performance of simple operations. The triangular type is derived from the diagonal, so the matrix is also square. The triangular form of the matrix is divided into vernetroyerinpy and negativly.

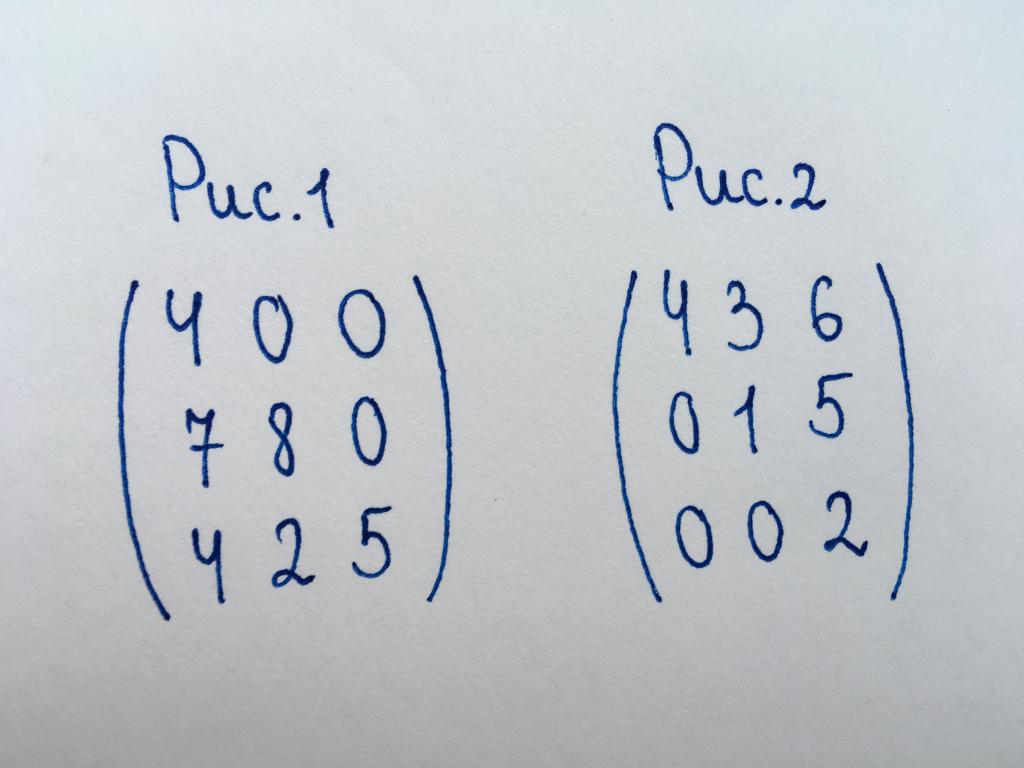
In vernerable matrix (Fig. 1) only items that are above the main diagonal are set to zero. The components of the diagonal of the matrix, arranged below it, contain numerical values.

In Nizhneserginsky (Fig. 2), on the contrary, the elements located in the lower part of the matrix is equal to zero.

Step matrix

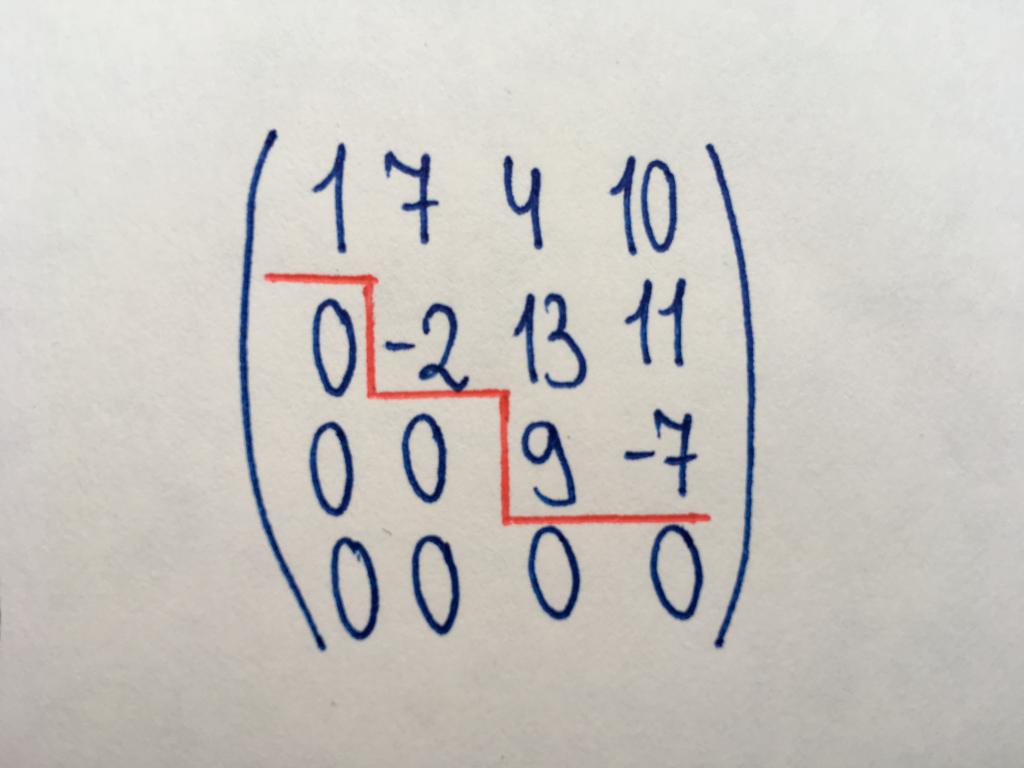
Required to find the rank of a matrix and elementary operations on them (along with the triangular type). The step matrix is so named because it contains the characteristic "steps" of zeros (as shown in the figure). In the stepped type forms a diagonal of zeros (not necessarily important), and all elements below the diagonal have the value zero. The mandatory condition is the following: if the step matrix there is a null-string, the other strings below it, also do not contain numerical values.

Thus, we considered the most important types of matrices needed to work with them. Now let's deal with the task of converting a matrix into the desiredform.

Reduction to triangular form

How to transform the matrix to a triangular form? Most often the tasks necessary to transform a matrix to triangular form, to find its determinants, otherwise called the determinant. Performing this procedure, it is important to "save" the main diagonal of the matrix because the determinant of the triangular matrix that is equal to the product of the components of its main diagonal. Let me remind you also alternative methods of finding the determinant. The determinant of a square is with the aid of special formulae. For example, you can use the method of triangle. For the other matrices using the method of decomposition by row, column, or elements. It is also possible to apply the method of minors and algebraic additions of the matrix.

Analyze in Detail the process of bringing a matrix to triangular form on the examples of some job.

Task 1

Need to find the determinant of the matrix is presented, using the method of bringing it to a triangular form.

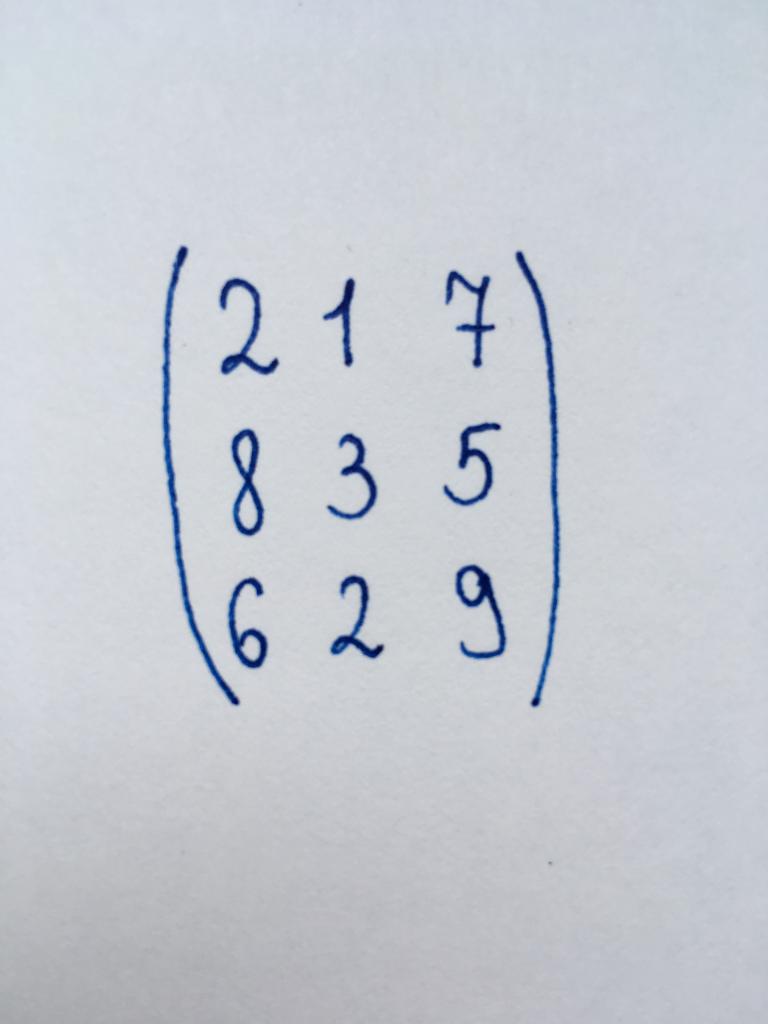
Given us the matrix is a square matrix of the third order. Consequently, to transform it into a triangular shape, we need to turn to zero the two components of the first column and one second component.

To bring it to triangular form, we begin the transformation from the lower left corner of the matrix with the number 6. To turn it to zero, we multiply the first row by three and subtract it from the last row.

Important! The top row is not changed and remains the same as in the original matrix. To write a string that is four times a large source is not necessary. But string values, the components of which you need to pay in zero, constantly changing.

Let us Further the following value - element of the second row of the first column, 8 in number. Multiply the first row by four and subtract it from the second row. Will receive a zero.

Leaving only the last value is the element of the third row of the second column. This number (-1). To turn it into zero, first subtract the second row.

Perform test:

DetA = 2 x (-1) x 11 = -22.

So, the answer to job: -22.

Exercise 2

Need to find the determinant of the matrix by bringing it to a triangular form.

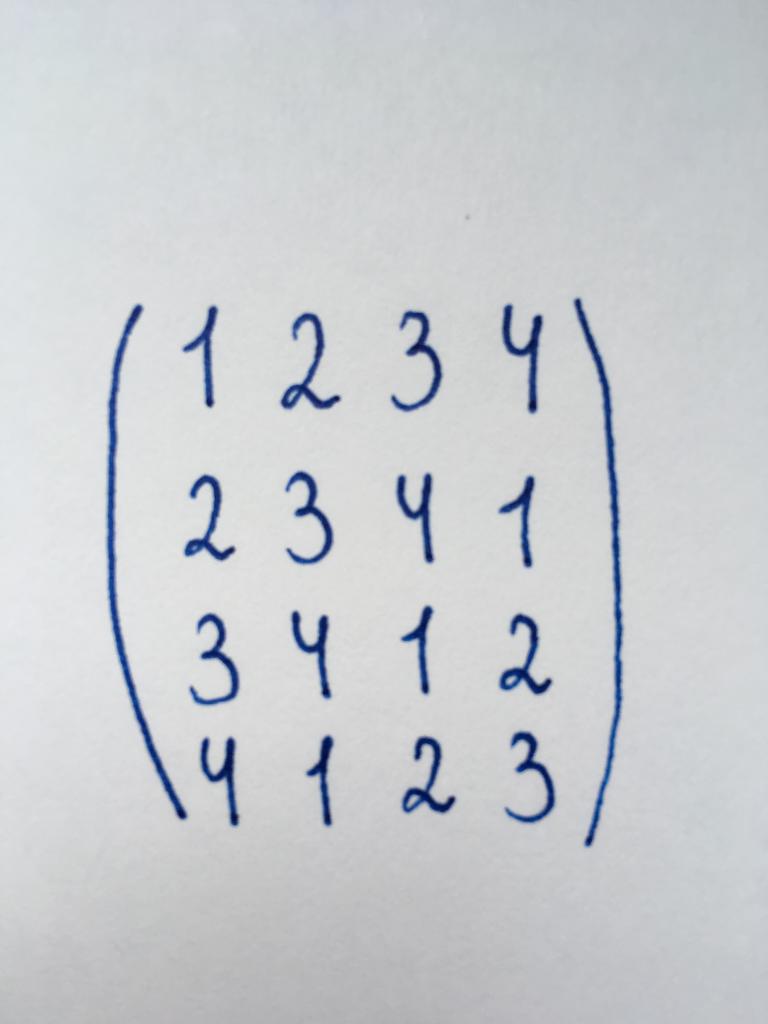
The matrix belongs to the square type and is the matrix of the fourth order. So, you need to pay in zero three components of the first column, the two components of the second column and one of the third component.

Let's Start bringing it with the element in the lower left corner with the number 4. We need to reverse this number to zero. Most convenient to do this by multiplying the top four string, and then subtract it from the fourth. Write the result of the first stage of the conversion.

So, the component of the fourth line converted to zero. Move to the first item in the third line, for number 3. Perform the same operation. Multiply by three the first row, subtract it from the third row and record the result.

Next we see the number 2 in the second line. Repeat the operation: multiply the top row by two and subtract it from the second.

We were able to pay to zero all the components of the first column of this square matrix, with the exception of the number of 1 - elements of the main diagonal that do not require conversion. It is now important to maintain the zeros, so we will convert to rows not columns. Go to the second column presents the matrix.

Again, start with the lower part - with element of the second column of the last row. This number (-7). However, in this case it is more convenient to start with a number (-1) - element of the second column of the third row. To turn it to zero, subtract from the third row to the second. Then multiply the second row by seven and subtract it from the fourth. We got a zero instead of an element located at the fourth row of the second column. We now turn to the third column.

In this column, we need to pay to zero only one number - 4. It is easy to do: just add to the last line of the third and we see we need the zero.

After all the transformations we have brought the proposed matrix to a triangular form. Now, to find its determinants, it is only necessary to perform a multiplication of the resulting elements of the main diagonal. Received: DetA = 1 x (-1) x (-4) x 40 = 160. Therefore, the solution is the number 160.

So now the question of reduction of a matrix to triangular form you please.

Bringing to mind the step

With the elementary operations on matrices stepped appearance is less "popular" than triangular. It is most commonly used for finding rank of a matrix (i.e. the number of its nonzero rows), or to define linear dependent and independent rows. However, the stepped form of the matrix is more versatile as it is suitable not only for square type, but for all the others.

To bring the matrix to speed mind, you first need to find its determinants. For this fit the above-mentioned methods. The goal of finding determinants is as follows: find out whether you can convert it into a step matrix. If the determinant is greater than or less than zero, then you can safely proceed to the job. If it is zero, to cast the matrix to speed mind will not work. In this case, you need to check if there are any mistakes in the record or in the transformation matrix. If such inaccuracies no jobit is impossible to solve.

Consider how to transform the matrix to speed mind the examples of multiple jobs.

Task 1. to Find the rank of this matrix.

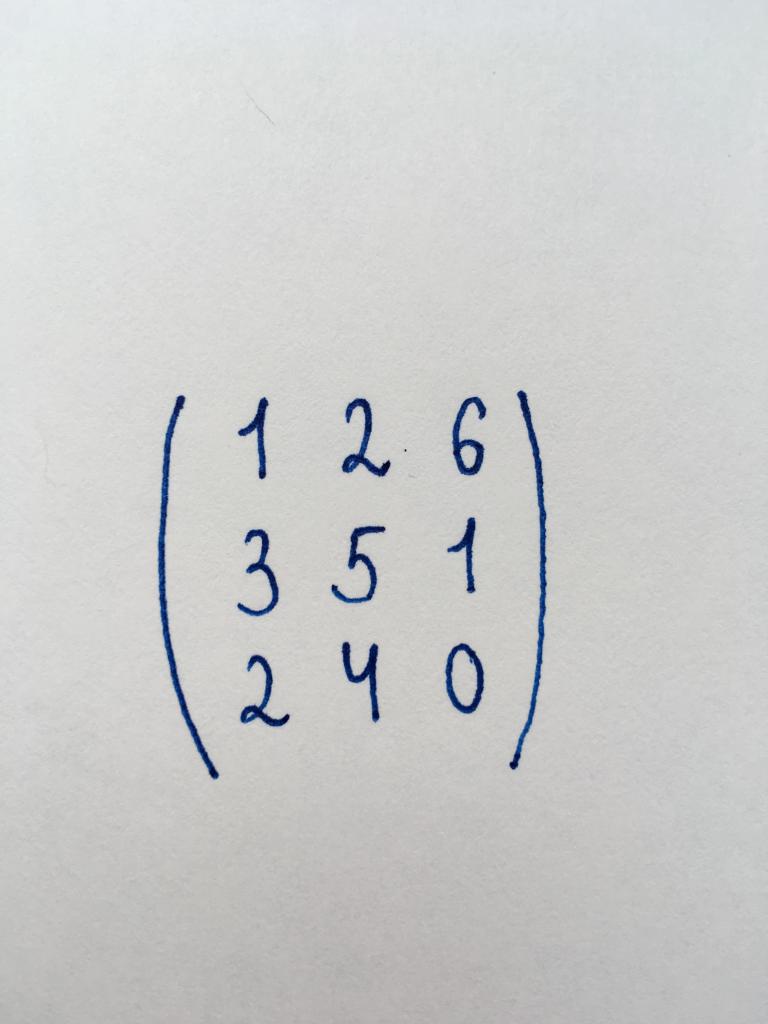
Before us is a square matrix of the third order (3x3). We know that to find the grade you must bring it to a speed mind. So first we need to find the determinant of the matrix. We use the method of triangle: DetA = (1 x 5 x 0) + (2 x 1 x 2) + (6 x 3 x 4) - (1 x 1 x 4) - (2 x 3 x 0) - (6 x 5 x 2) = 12.

Determinants = 12. It is greater than zero, then the matrix can lead to speed mind. Start its transformation.

Start with element to the left column of the third row, numbers 2. Multiply the top row by two and subtract it from the third one. Thanks to this operation as the needed element and the number 4 element of the second column of the third row is turned to zero.

Next, draw to zero the element of the second row of the first column is the number 3. To do this multiply the top row by three and subtract it from the second.

We can see that the cast was formed of a triangular matrix. In our case, to continue with the conversion is impossible, as the other components will not be able to pay to zero.

So, we conclude that the number of rows that contain numeric values in the matrix (or rank) is 3. Answer to job: 3.

Task 2. to Determine the number of linearly independent rows of this matrix.

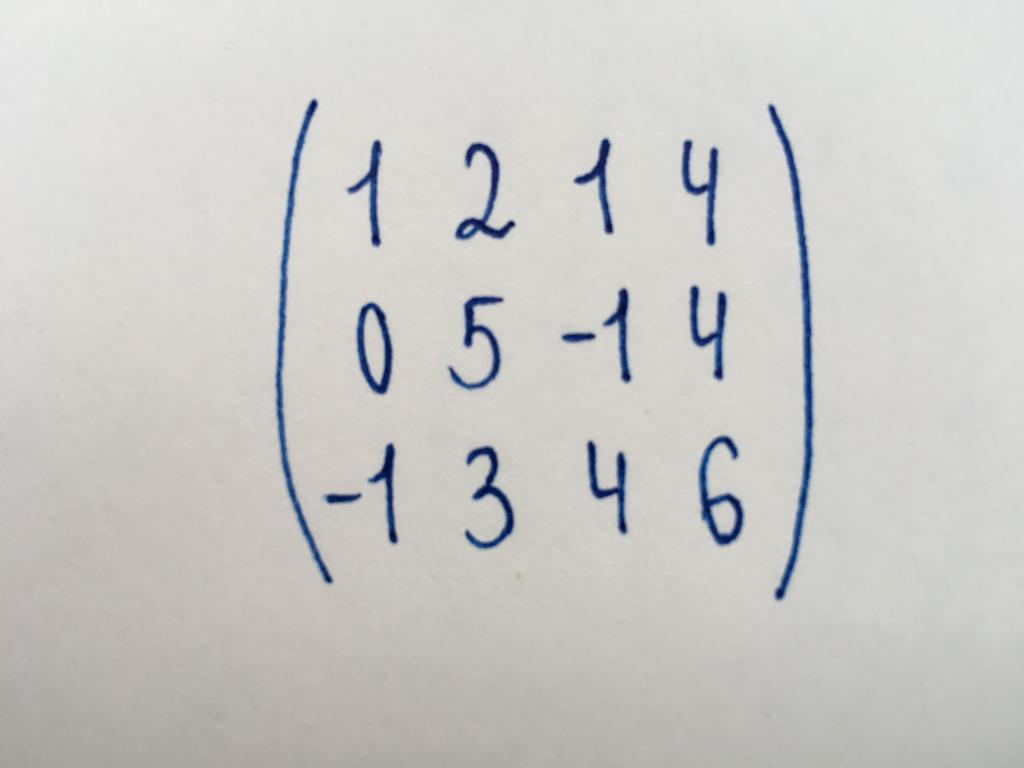
We need to find strings that are not any transformations to draw to zero. In fact, we need to find the number of nonzero rows or the rank of the represented matrix. To do this, perform its simplification.

We see a matrix that does not belong to the square type. It has dimensions 3x4. Let's start the cast with the item's lower left corner - the number (-1).

We Add the rst row to the third. Next, subtract the second to draw the number 5 to zero.

Further conversion is impossible. Hence, we conclude that the number of linearly independent rows in it, and the answer to the task 3.

Now the reduction of a matrix to speed sight is an impossible task.

For examples of the jobs we discussed the reduction of a matrix to triangular form, and a stepped appearance. To make the desired values zero matrix tables, in some cases you may need to show imagination and to correctly convert these columns or rows. Wish you success in mathematics and in working with matrix!